If you’d asked ten-year-old me how to do multiplication algorithms, I would have looked at you like you had six eyes. Algorithms… plural? I only knew of one: the standard algorithm. I’d memorized the steps after months of agonizing about how I was ‘bad at math’ while crying over the Costco workbook my mom had bought because I needed “extra practice”. But I had no idea why the standard algorithm worked. Every time I asked someone why that zero went in the second line or why you multiplied the ones first, I was told – “that’s just the way it works.”
Well… that’s not “just the way it works”. There’s a really good reason that the standard algorithm for multiplication works the way that it does. Perhaps if I knew why it worked, I would have been able to figure out how to do it much sooner. And maybe I would have hated math a little less.
I tried not to bring a negative attitude about math into my practice when I first started teaching, but it slipped in. I didn’t see the point in teaching my students how to do multiplication algorithms more than one way. The way I saw it, more algorithms just meant more opportunities for confusion. That assumption was based on another assumption: that math in and of itself was confusing.
It shouldn’t be.
Thankfully, after listening to the advice of some phenomenal teachers, I finally started introducing conceptual multiplication strategies to my students. And guess what? They’d been right all along; I should have been doing it the whole time.

Why should we teach students how to do multiplication algorithms multiple ways?
Once I started teaching how to do multiplication algorithms more than one way, my students were way more successful! They were also much more confident and could do multi-digit multiplication in their heads more efficiently because they had a better understanding of how important it was to break up numbers into their place value parts.
Now that I’ve seen the light, I teach my students how to do multiplication algorithms four ways.
Four different multiplication algorithms
The Area Model of Multiplication a.k.a. The Box Method
The area model of multiplication is the multiplication algorithm that I like to teach first. I usually go into multiplication after reviewing place value with whole numbers and extending that learning to introduce decimals; this multiplication algorithm makes the connections to place value very explicit.
Pros:
· This method makes each step in the multi-digit multiplication process very clear.
· It simplifies the multiplication calculations students are working on because every number that isn’t a single digit is going to end in at least one zero.
· The connection between multi-digit multiplication and place value is made very clear from the moment the problem is set up.
· I’ve seen lots of students who really struggle with the other multiplication algorithms have great success with this one.
· Students who struggle to keep track of the regrouping required when adding the partial products can break it up into several addition equations by adding the sums of each column separately and then adding those together.
Cons:
· It’s more time-consuming than the standard algorithm.
· It takes up more space on the page than the standard algorithm.
· Guardians may be less familiar with this model and may struggle to help their children with it.
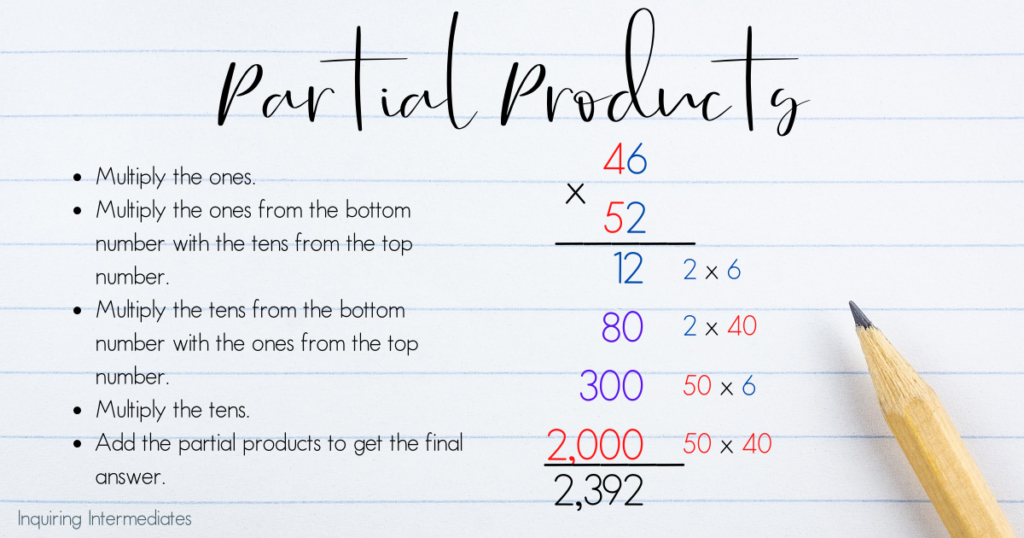
Multiplication with Partial Products
Doing multiplication with partial products is basically putting the area model in the standard algorithm’s clothing… In other words, the process is the same as in the area model, but it looks more like the standard algorithm.
Pros:
· This method makes each step in the multi-digit multiplication process very clear.
· Students who already know the area model of multiplication can easily solve problems using the partial products method.
· This method can be used with worksheets designed to be used with the standard algorithm.
Cons:
· It takes up more space than the standard algorithm.
· When you start multiplying large numbers, you do a ridiculous amount of adding (there’s one line of partial products for each digit in the problem), so students can easily make mistakes in the adding portion of the solution.
· Students are required to recognize and remember how place value impacts a digit’s value, and the way the problem is setup doesn’t provide as clear of a reminder as in the area model.
· Many guardians are unfamiliar with the partial products method and may not be immediately able to help their children with
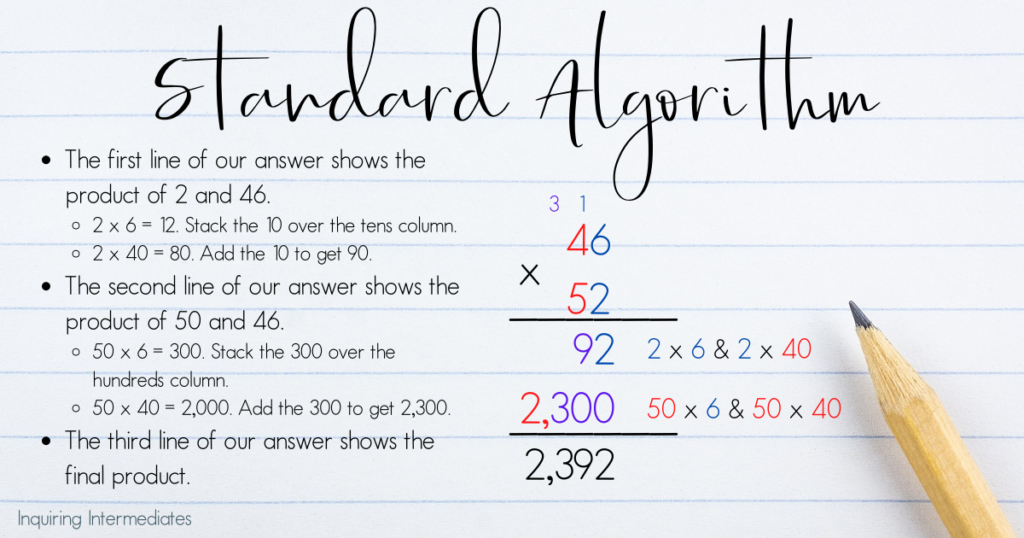
The Standard Algorithm for Multiplication
The standard method is the one that I grew up with and that was most commonly taught in North America when I was a kid. It’s also the one that research in the 1970s and 1980s found students didn’t actually understand, although I figure that’s probably more of a teaching issue than an algorithm problem.
Pros:
· It can be much faster than the area model or the partial products method.
· Guardians who grew up in North America are most likely to be familiar with this multiplication algorithm, which makes it easier to help their children at home.
· There is less adding required than in the area model or partial products method.
Cons:
· Students are required to recognize and remember how place value impacts a digit’s value, and the way the problem is set up doesn’t provide as clear of a reminder as in the area model. This is the case while multiplying the pieces of the factor and when writing the partial products.
· There is the possibility of regrouping being needed for each step, which gives students another thing to remember.
The Line Method of Multiplication
Some people call the line method of multiplication The Japanese Multiplication Trick, but I can’t seem to find any definitive proof that it is specifically from Japan. Plus, it really isn’t a trick. A hack? Maybe. But not a trick!
Pros:
· Once students learn how to use the line method of multiplication, they can solve problems super quickly.
· Students who subitize well are especially fast with this method.
· The connection between multi-digit multiplication and place value is made very clear from the moment the problem is set up.
Cons:
· The fact that the initial set up involves no numbers can make the line method super overwhelming at first.
· Many guardians are unfamiliar with the line model and may not be immediately able to help their children with it.
Grab resources for how to do multiplication algorithms four ways!
If you’re looking to introduce a new strategy to your students, don’t reinvent the wheel! You can find print-and-go guides and worksheets here.
Tips for teaching multiplication algorithms:
·
Start with the conceptual multiplication
strategies first… even if they’re not the most efficient. Once students
understand how multi-digit multiplication works, they’ll have a much easier
time understanding the more efficient algorithms. This is the order I go and why
I do it that way:
o
Area model: helps students understand what multi-digit
multiplication really is.
o
Partial products method: the steps are the same,
but the setup is different and more like the standard algorithm.
o
Standard algorithm: it’s essentially a short-cut
version of the partial products method. It’s also more efficient than the first
two.
o
Line method of multiplication: once you’ve
learned it, it can be the fastest multiplication algorithm by far. However, it can
look a bit abstract at first.
·
Practice, practice, practice! Although I’m all
about students getting to pick the methods that work well for them, few things
work well without practice.
·
Get families on board! Have students teach these
algorithms to their family members. Without prompting, I know several of my
students went home and excitedly taught the line method of multiplication to
their parents.
How do we help families who don’t know how to do multiplication algorithms the way we do them at school? How are they going to support their students?
Here are three ways I try to address this:
1. On the front of the first worksheet, students are given for each new algorithm, print a step-by-step guide. When students are finished their first page, mark it together and send it home so families can reference the guide and all the questions students have already completed. I have guides and worksheets for these four multiplication algorithms here.
2. Record simple videos and share them with families. My videos are relatively low-tech. I just screen-record the notes app on my school iPad, writing with a stylus, and narrating as I go. This year, several students volunteered to create videos in their home languages. I’m so excited about the opportunities this will create for more families to explore these algorithms with their students! If you don’t have the technology to make your own videos, find one online and send the link.
3. Remember that one reason we teach more than one algorithm is so that students can pick which works for them. Maybe students don’t actually need to be totally comfortable with all four algorithms (personally, I always find myself slipping up with the partial products method). If their families can teach them a different way that they understand, let them use it!
Activities for teaching students how to do multiplication algorithms:
· Start with a demonstration. Do an example and then work as a class or in small groups to do more practice equations on whiteboards or scrap paper.
· Co-create an anchor chart that shows how to do multiplication algorithms several ways.
· Worksheets with a step-by-step guides that students can take home.
· Paired practice. Have students pick partners that they feel are as confident with this method as they are. They can give each other questions to solve on whiteboards and check one another’s work. This allows students to give themselves problems that meet them where they are at. It also allows you to target the pairs you give extra support to.
· Peer tutors and small groups. While you work with small groups, some students can work independently. There will likely be students who have already shown they know the method you are learning; they can float around as peer tutors and help the kids who are working independently.
· Equation pass-arounds. Students work in groups of three or four to pass around and complete equations one step at a time.
· Compare the algorithms. Once you’ve learned more than one algorithm, be sure to make the parallels explicit. Better yet, have students work in small groups to come up with similarities or differences. You can also have volunteers come up to the board and demonstrate how the processes are similar or different as they solve the same problem simultaneously, but with different multiplication algorithms.
Grab these supporting resources!
Looking to teach your students how to do multiplication algorithms four ways? For each of the algorithms mentioned in this post, I have a resource with a step-by-step guide, ten scaffolded worksheets, and a complete answer key!
· 4 Strategies for Multiplication Bundle (includes the four resources below)
· Area model of multiplication / Box method
· Multiplication with partial products